Algèbre linéaire et bilinéaire I (2MA221)

Voir aussi la page de Yves Coudène.

Polycopié du cours (par Yves Coudène)

Feuilles d'exercices: TD 1, TD 2, TD 3, TD 4, TD 5

Partiel du 19 novembre: énoncé, corrigé

Avancement du cours

Cours 1 (24 septembre)

• Définition d'une matrice (Chapitre 1, 1.1).
• Rang d'une matrice (Chapitre 1, 1.2)
• Définition d'une matrice échelonnée (Chapitre 1, 2.1)
• Propriétés (Chapitre 1, 2.2)
• Mise sous forme échelonnée (Chapitre 1, 3.1)
• Résolution de systèmes linéaires (Chapitre 1, 3.2)
• Interprétation matricielle (Chapitre 1, 3.3)
• Applications (Chapitre 1, 3.4)

Cours 2 (1 octobre)

• Définition d'une forme linéaire (Chapitre 2, 1.1)
• Noyau d'une forme linéaire (Chapitre 2, 1.2)
• Base duale (Chapitre 2, 1.3)
• Le cas de $\mathbb{R}^n$ (Chapitre 2, 1.4)

Cours 3 (8 octobre)

• Définition d'une forme bilinéaire (Chapitre 2, 2.1)
• Représentation matricielle (Chapitre 2, 2.2)
• Changement de base (Chapitre 2, 2.3)
• Rang et noyau (Chapitre 2, 2.4)
• Définition d'une forme quadratique (Chapitre 2, 3.1)
• Signature (Chapitre 2, 3.2)

Cours 4 (15 octobre)

• Réduction des formes quadratiques (Chapitre 3, 3.3)
• Base orthogonale (Chapitre 3, 3.4)

Cours 5 (22 octobre)

• Interprétation matricielle (Chapitre 3, 3.5)
• Étude de la signature (Chapitre 3, 3.6)
• Coniques (Chapitre 3, 4.1)

Cours 6 (29 octobre)

• Quadriques (Chapitre 2, 4.2)
• Définition d'un produit scalaire (Chapitre 3, 1.1)
• Norme euclidienne (Chapitre 3, 1.2)
• Aire, longueur et angle (Chapitre 3, 1.3)

Cours 7 (5 novembre) (notes)

• Aire, longueur et angle (Chapitre 3, 1.3)
• Projection orthogonale (Chapitre 3, 2.1)

Cours 7 (3 décembre) (notes)

• Projection et symétrie orthogonale (Chapitre 3, 2.1)
• Procédé d'orthonormalisation de Gram-Schmidt (Chapitre 3, 2.2)
• Définition d'une isométrie (Chapitre 3, 3.1)
• Groupe orthogonale (Chapitre 3, 3.2)
• Isométries du plan euclidien (Chapitre 3, 3.3)

Cours 9 (10 décembre) (notes)

• Définition du déterminant (Chapitre 4, 1.1)
• Calcul de déterminants (Chapitre 4, 1.2)
• Propriétés des déterminants (Chapitre 4, 1.3)
• Déterminant d'une application linéaire (Chapitre 4, 1.4)
• Somme de sous-espaces vectoriel (Chapitre 4, 2.1)
• Valeurs propres et vecteurs propres (Chapitre 4, 2.2)

Cours 10 (17 décembre) (notes)

• Diagonalisation (2.3)
• Interprétation matricielle (2.4)
• Cas des valeurs propres distinctes (2.5)
• Adjoint d'une application linéaire (3.1)
• Diagonalisation des applications autoadjointes (3.2)

Fondements d'analyse et algèbre (2MA205)

Avancement du cours

Cours 1 (21 septembre)

• Rappels sur les relations: ordre partiel, ordre total, équivalences
• Construction des entiers relatifs, de l'opération de somme, de l'opposé.

Cours 2 (26 septembre)

• Fin de la costruction des entiers: multiplication et ordre
• Construction des nombres rationnels et ses opérations.

Cours 3 (2 octobre)

• Construction des nombres réels.
• Somme, multiplication, division de nombres réels.
• Ordre sur les réels. Valeur absolue.

Cours 4 et 5 (9 et 16 octobre)

• Convergence de suites de Cauchy réelles.
• Propriété de la borne supérieure.
• Représentation en base $N$ d'un nombre réel..

Cours 6 (23 octobre)

• Cardinalité. Exemples: $\mathbb{N}$, $\mathbb{Z}$, $\mathbb{Q}$.
• Théorème de Cantor-Schröder-Bernstein.
• Cardinalité de l'ensemble des parties.
• Cardinalité de $\mathbb{R}$.

Cours 7 (30 octobre)

• Nombres irrationnels, algébriques, transcendants.
• Division euclidienne des polynômes.
• Idéaux dans l'anneaux des polynômes.
• Nombre de racines d'un polynôme.
• Cardinalité des algébriques et des transcendents.

Cours 8 (6 novembre) (notes)

• Théorème de Liouville.
• Nombres de Liouville.

Cours 9 (23 novembre) (notes)

• Anneau, idéaux, anneaux intègres, corps. Exemples.
• Anneau quotient.
• Idéaux premiers.
• Polynômes irréductibles et premiers. Factorisation unique.
• Extension de corps reletive à un polynôme irréductible.

Projets pour le cours (notes)

1. Transcendence de $e$ et de $\pi$. (Bouzar-Sun)
2. Théorème de Lindemann-Weierstrass.
3. Le théorème d'Apéry : irrationalité de $\zeta(3)$ (Acard-Bouallouche)
4. Les nombres $p$-adiques : construction et méthodes de Newton. (Hadj Slimane-Bessonneau)
5. Entiers de Gauss. (Mansour Bourassine-Kurdyk)
6. Réciprocité quadratique
7. Nombre de polynômes irréductibles sur un corps fini. (Madi-Saïd-Otmane)
8. La fonction $\zeta$ de Riemann et ses valeurs aux entiers pairs. (Moreau-Venedittan)
9. Polynômes cyclotomiques et construction de polygônes réguliers à la règle et au compas. (Blasco-Braun)
10. Équation de Pell-Fermat (Devinat-Saci)

Elliptic curves

Lecture 1 (November 3rd) (notes)

• Elliptic functions ([Silverman, Chapter VI, §2 and §3 up to Theorem 3.5])

Weierstrass' $\wp$ and $\wp'$.

Lecture 2 (November 4th) (notes)

• Proof of the embedding Theorem for complex elliptic curves. ([Silverman, Chapter VI, Theorem 3.5])
• Interpretation of the embedding via Riemann-Roch ([Reyssat, Chapter X, § 1])
• Group morphisms between elliptic curves ([Silverman, Chapter VI, § 4])
• The set of equivalences classes of elliptic curves ([Serre, Chapter VII, § 2.2])
• Action of $\textrm{SL}_2(\mathbb{Z})$ on the upper half plane ([Serre, Chapter VII, § 1])

The action of $z \mapsto z+1$	The action of $z \mapsto -1/z$

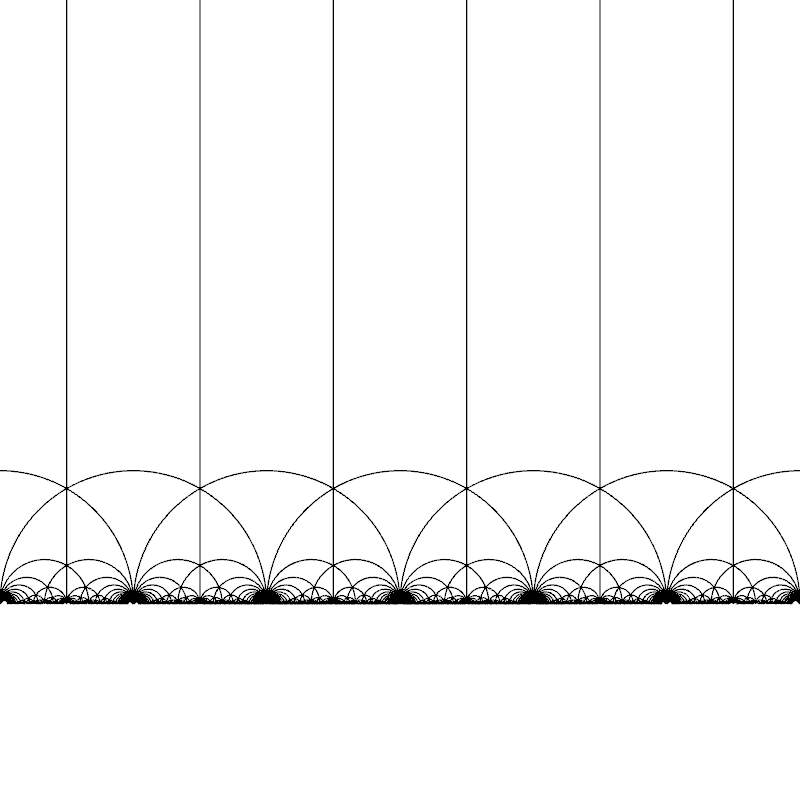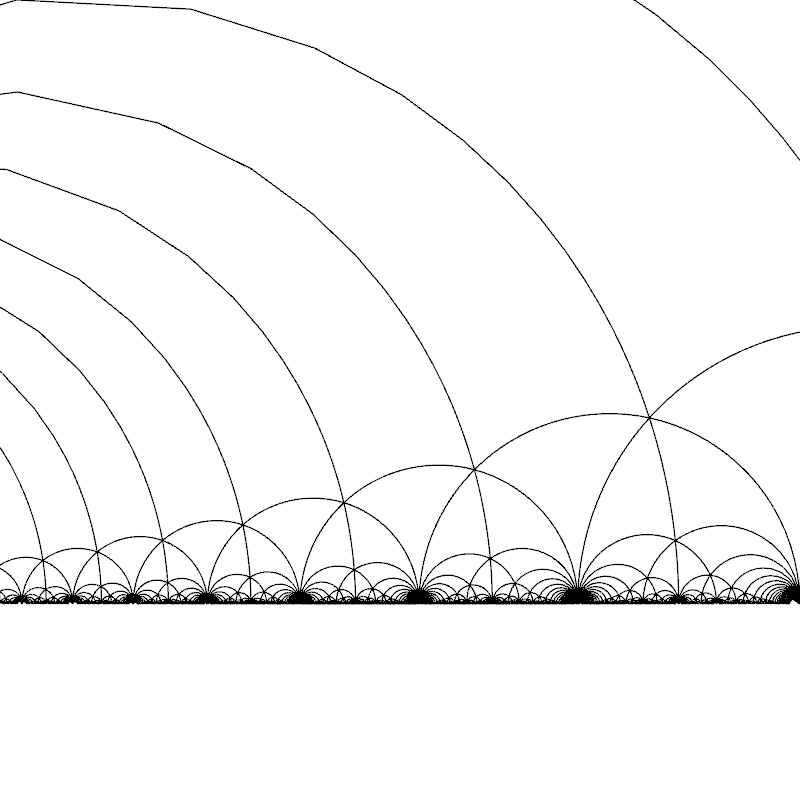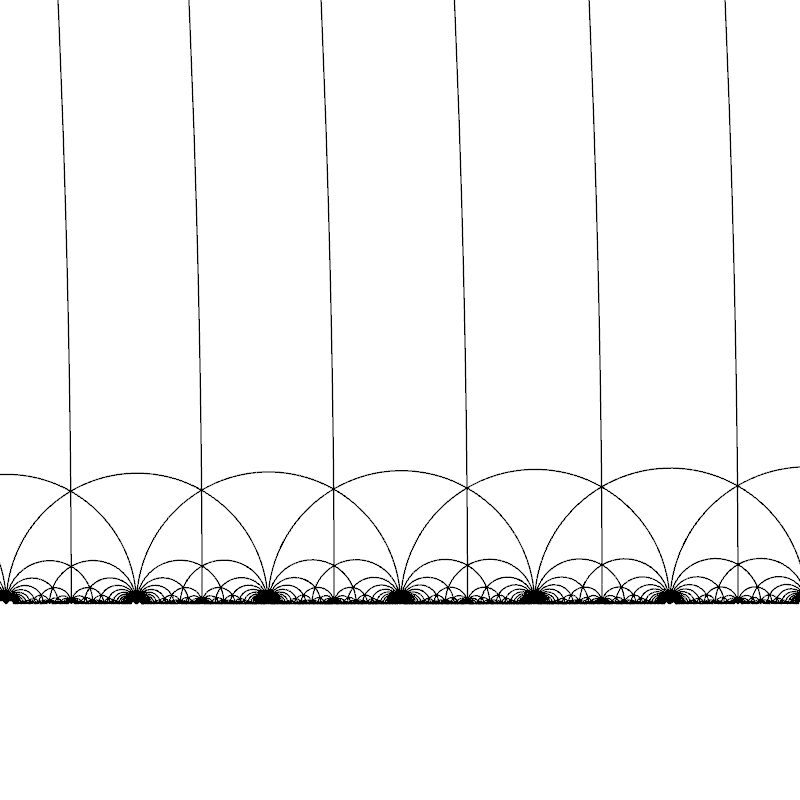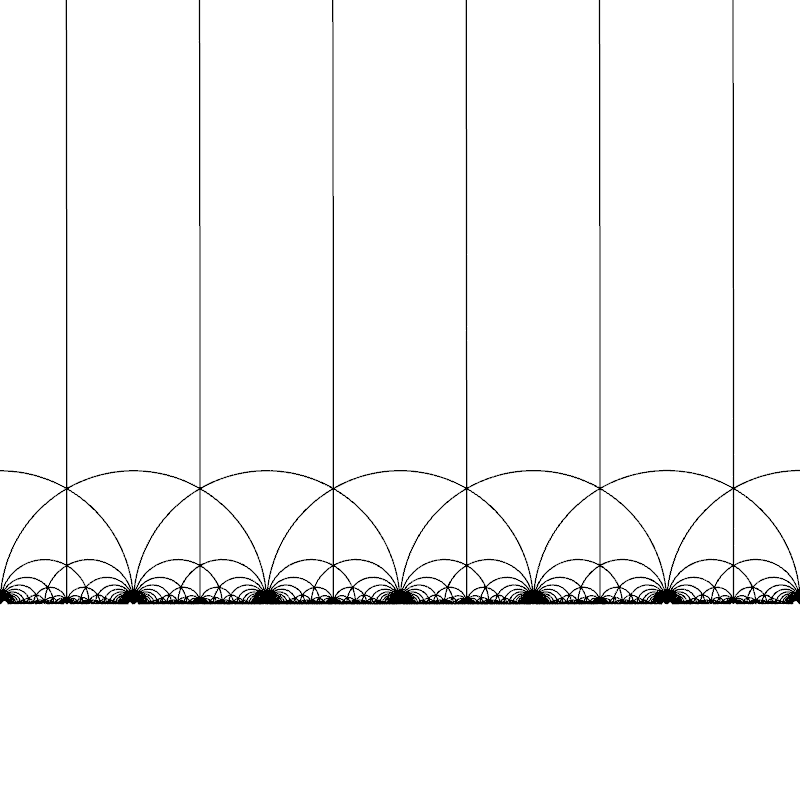Lecture 3 (November 6th) (notes)

• The Eisenstein series are modular forms of weight $2k$ ([Serre, Chapter VII, § 2.3]).
• The $j$-invariant of an elliptic curve ([Serre, Chapter VII, § 3.3]).
• Formula for the order of vanishing of a modular form of weight $2k$. ([Serre, Chapter VII, Theorem 3])
• The $j$-invariant is a bijection $\mathbb{H}/\textrm{SL}_2(\mathbb{Z}) \stackrel{\sim}{\rightarrow} \mathbb{C}$ ([Serre, Chapter VII, § 3.3])
• Torsion group of a complex elliptic curve ([Lang, Chapter 2, § 1], [Silverman, Chapter VI, Proposition 5.4])
• Summation formula for $\wp$ ([Lang, Chapter 1, § 3])
• Endomorphisms and automorphisms of a complex elliptic curve. ([Silverman, Chapter VI, Theorem 5.5])

Lecture 4 (November 10th) (notes)

• Normal, regular, discrete valuation, Dedekind rings. ([Dat, p. 10-12]; Stacks)
• Definition of an algebraic variety.

Lecture 5 (November 11th) (notes)

• Algebraic curve associated with a field of transcendance degree 1. ([Dat, p. 13-14])

Lecture 6 (November 13th): "Exercises" (notes)

• Artinian rings (Stacks)
• Degree formula for maps between algebraic curves

Lecture 7 (November 17th) (notes)

• Morphisms between smooth projective curves. ([Dat, p. 13-16])
• Divisors. ([Dat, p. 16-18])

Lecture 8 (November 18th) (notes)

• Kähler differential forms. ([Dat, § 2.3.2], [Liu, § 6.1.1])

Lecture 9 (November 20th) (notes)

• Divisor of a meromorphic differential form, Hurwitz formula. ([Dat, -. 21-22])
• Riemann-Roch (without proof). ([Dat, § 2.3.4])
• Embeddings associated with a divisor. ([Dat, § 2.2.3 and 2.3.5])

Lecture 10 (November 24th) (notes)

• Proof of the embedding theorem. ([Dat, § 2.2.3 and 2.3.5])
• Embedding of an elliptic curve. Weierstrass equation. ([Dat, Théorème 3.1.3 (i)])
• Smooth projective plane curves

Lecture 11 (November 25th) (notes)

• Genus of a smooth plane cubic.
• Group law on a smooth plane cubic.
• 2-torsion points (in characteristic different from 2)
• Isomorphism $E(k) \rightarrow \mathrm{Pic}^0(E)$

Lecture 12 (December 1st) (notes)

• Group law is an algebraic map. ([Dat, § 3.1.4])
• Frobenius twists and inseparable morphisms. ([Dat, § 2.4.3])
• Isogenies. ([Dat, § 3.2.1])
• Multiplication by $m$. ([Dat, § 3.2.2])
• Invariant differentials. ([Dat, § 3.3])

Lecture 13 (December 2st) (notes)

• Proof of [Dat, Theorem 3.3.1]
• Quotient by a finite subgroup. ([Dat, Lemma p. 35])
• Dual isogenies. ([Dat, § 3.2.3])
• Definition of Weil's pairing. ([Dat, § 3.4.1])

Lecture 14 (December 4th) (notes)

• 2-torsion points in characteristic 2.
• Frobenius.
• Automorphisms.

Lecture 15 (December 8th) (notes)

• End of proof of [Dat, Proposition 3.4.2]
• An isogeny and its dual are adjoint with respect to Weil's pairing. ([Dat, Proposition 3.4.3])
• The dual of the sum is the sum of the duals. ([Dat, Theorem 3.2.4])
• $\mathrm{deg}([m]) = m^2$, at long last! ([Dat, Corollary 3.2.5])
• The degree is a positive definite quadratic form. ([Dat, Corollary 3.2.6])
• Hasse's bound of $\mathbb{F}_q$-rational points. ([Dat, Theorem 3.2.7])
• Tate module. ([Dat, § 3.5.1-2])

Lecture 16 (December 9th) (notes)

• End of proof of [Dat, Theorem 3.5.3]
• Endomorphism ring. ([Dat, § 3.6.1, 3.6.2, Lemma and Proposition p. 52])
• Weierstrass equation (reprise). ([Dat, § 3.7.1])
• Group law on singular cubics.([Dat, § 3.7.3])
• Over non-Archimedean fields (only statements). ([Dat, § 3.8.1, 3.8.3])
• Statement of Mordell's Theorem. ([Dat, § 3.9])

Lecture 17 (December 11th) (notes)

• Characterization of supsersingular curves.
• Examples of supersingular curves.
• The $j$-invariant.

Lecture 18 (December 15th) (notes)

• Counting of supsersingular curves.
• Singular Weierstrass equation.

References

• J.-F. Dat, Introduction à l'arithmétique des courbes elliptiques
• N. Bergeron, A. Guilloux, Introduction aux surfaces de Riemann
• O. Forster, Lectures on Riemann surfaces
• J. Milne, Modular Functions and Modular Forms
• J. H. Silverman, The Arithmetic of Elliptic Curves
• E. Reyssat, Quelques aspects des Surfaces de Riemann (pdf here)
• J.-P. Serre, Cours d'arithmètique (also translated in english, A course in Arithmetic)
• S. Lang, Elliptic Functions
• Q. Liu, Algebraic Geometry and Arithmetic Curves

Some exercises and complements (in French)

• Bezout's Theorem (pdf and source of the exercises)
• Inflexion points (in arbitrary characteristics) and group law. (pdf and source of the exercises)
• Embeddings via Riemann-Roch. (pdf)